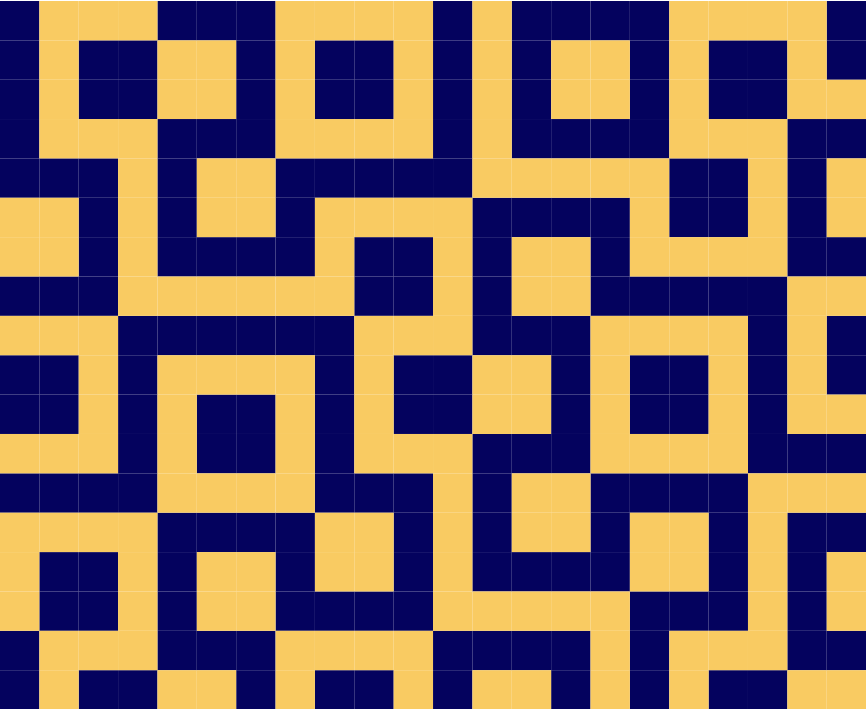
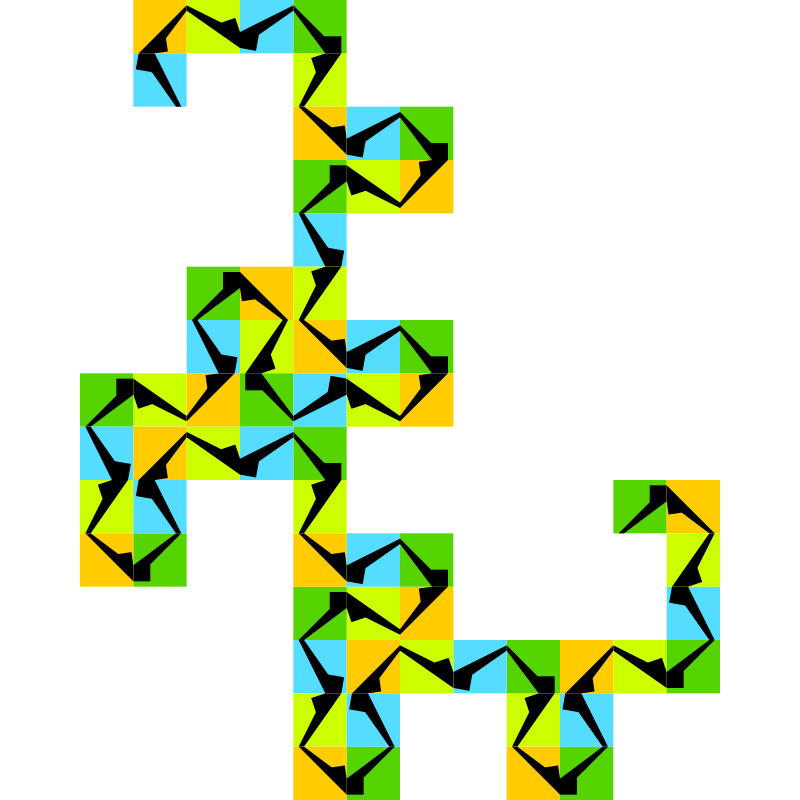Limitperiodic
A nonperiodic tiling is called limitperiodic, if it is the union of countably many periodic patterns (up to a set of zero density). It is quite easy to see that this can only be the case if the inflation factor (or a power of it) is an integer number. A tiling is limitperiodic if and only if it can be obtained through a cut and project scheme with p-adic internal space
The Gosper Curve is a FASS-curve which can be derived by a substitution tiling with one substitution rule and appropriate decorations.
The inflation factor $q$ is $sqrt(7)$.
Finite Local Complexity Polytopal Tiles Self Similar Substitution With Decoration Limitperiodic FASS_curve
The original Heighway Dragon Curve as described in [gar1967] , can be derived by a substitution tiling with one substitution rule and appropriate decoration. However, it is not a FASS-curve because it is not self avoiding. With the results in [pau2021] it is possible to derive a substitution tiling …
Finite Local Complexity Polytopal Tiles Self Similar Substitution With Decoration Limitperiodic FASS_curve
The Hilbert Curve is one of the earliest FASS-curves. The original algorithm in [hil1891] bases on one substitution rule and an additional rule which describes how the substitutes have to be connected. As briefly mentioned in [pau2021] it is also possible to create the Hilbert Curve by a …
Finite Local Complexity Polytopal Tiles Self Similar Substitution With Decoration Limitperiodic FASS_curve
The Monnier Trapezium and Diamond tiling uses two prototiles,
a trapezium and a rhomb. The inflation multiplier is $2$.
By changing the chiralities of the prototiles within the first level
supertiles several further variants can be derived.
Finite Local Complexity Polytopal Tiles Self Similar Substitution With Decoration Limitperiodic
The Nischke-Danzer-Deltoid 6-fold-2-2 was discussed and derived in [ND96] .
Polytopal Tiles Self Similar Substitution Finite Local Complexity Finite Rotations Nischke Danzer Deltoid Limitperiodic
The Peano Curve is one the earliest known FASS-curves. The original algorithm in [pea1890] bases on one substitution rule and an additional rule which describes how the substitutes have to be connected. As briefly mentioned in [pau2021] it is also possible to create the Peano Curve by a …
Finite Local Complexity Polytopal Tiles Self Similar Substitution With Decoration Limitperiodic FASS_curve
This Wanderer tiling is the first of an infinite series of substitution tilings by Joan Taylor based on paper-folding sequences. It uses a single square tile with four distinct rotations and two distinct reflections. Here we use colours to distinguish left-handed (brown) from right-handed (white) …
Limitperiodic Polytopal Tiles Rep Tiles Self Similar Substitution
This Wanderer tiling is one in an infinite series of substitution tilings by Joan Taylor based on paper-folding sequences. It uses a single square tile with four distinct rotations and two distinct reflections. Here we use colours to distinguish vertical (blue) from horizontal (ochre) tiles. In the …
Limitperiodic Polytopal Tiles Rep Tiles Self Similar Substitution