Heighway Dragon FASS-Curve Substitution Tiling
- FASS-curve
- Finite Local Complexity (FLC)
- Limitperiodic
- Polytopal Tiles
- Self-Similar Substitution
- With Decoration
Discovered by
Info
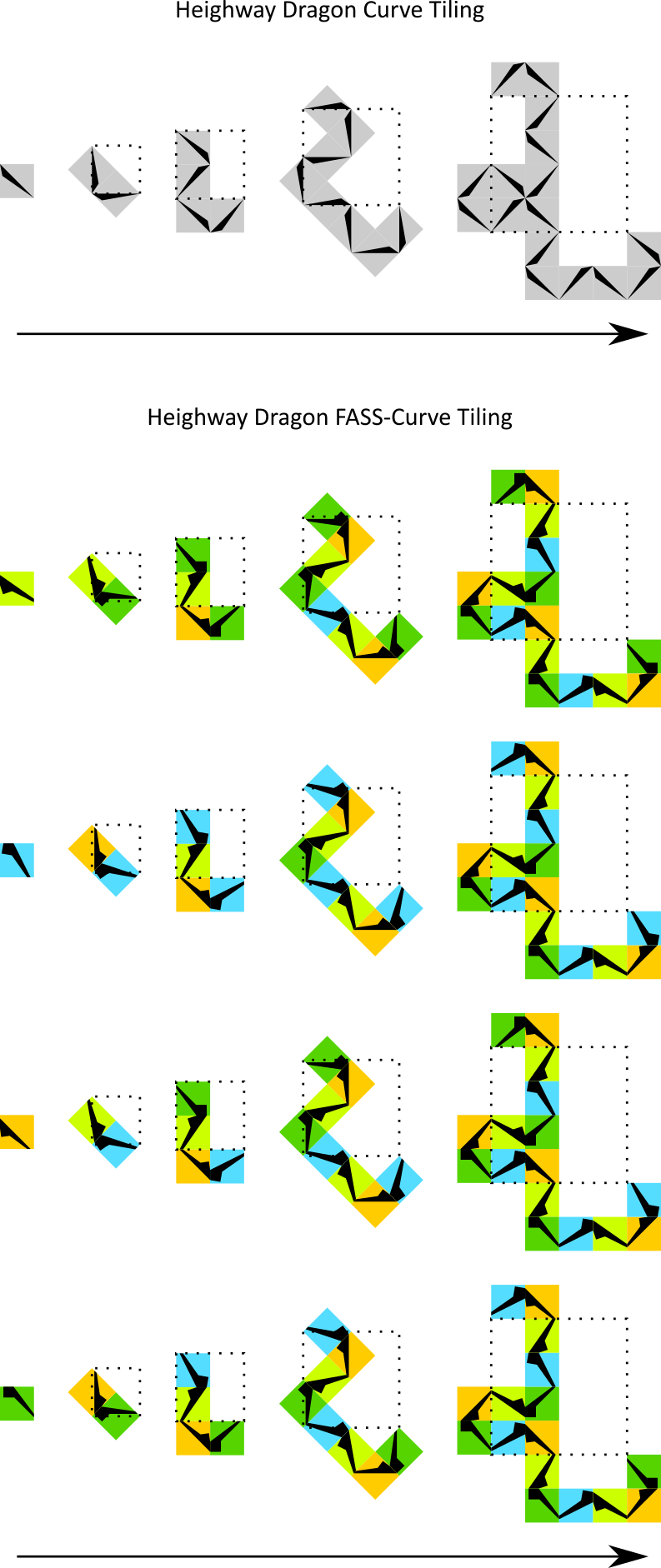
The original Heighway Dragon Curve as described in [gar1967]
, can be derived by a substitution tiling with one substitution rule and appropriate decoration.
However, it is not a FASS-curve because it is not self avoiding.
With the results in [pau2021]
it is possible to derive a substitution tiling which generates a Heighway Dragon FASS-Curve without disturbing self similarity.
In detail the decoration on the proto tile is shifted away from the corners in different ways. As a consequence of the combinatorics the number of substitution rules increases to four.
Furthermore the vertices of the FASS-curve are not preserved by the substitution rules.
The inflation factor $q$ is $\sqrt{2}$.
Substitution Rule
Patch
download vectorformat Heighway Dragon FASS-Curve Substitution Tiling
References
[gar1967]
Gardner, M
Mathematical Games
Scientific American
1967,
Volume 216, Issue 3,
124-127,
10.1038/scientificamerican0367-124
[Pau2021]
Pautze, S
Space-Filling, Self-Similar Curves of Regular Pentagons, Heptagons and Other n-Gons
Proceedings of Bridges 2021: Mathematics, Art, Music, Architecture, Culture
2021,
38,
157-164,
bridges2021-157.pdf