Kenyon's non FLC
Discovered by
Info
A simple substitution, yielding a tiling which is not of finite local complexity (flc). The substitution maps the single prototile, a unit square, to three columns of three squares each, where the third column is shifted by an irrational amount t. In higher iterates of the substitution, there are neighboured squares shifted against each other by t, 3t+t, 9t+3t+t,… mod 1. Since t is irrational, these sequence contains infinitely many values (mod 1), thus there are infinitely many pairwise incongruent pairs of tiles. Consequently, non-flc follows.
This example is not volume hierarchic. But it can be made into one, see Kenyon non-FLC (volume hierarchic).
Substitution Rule
Patch
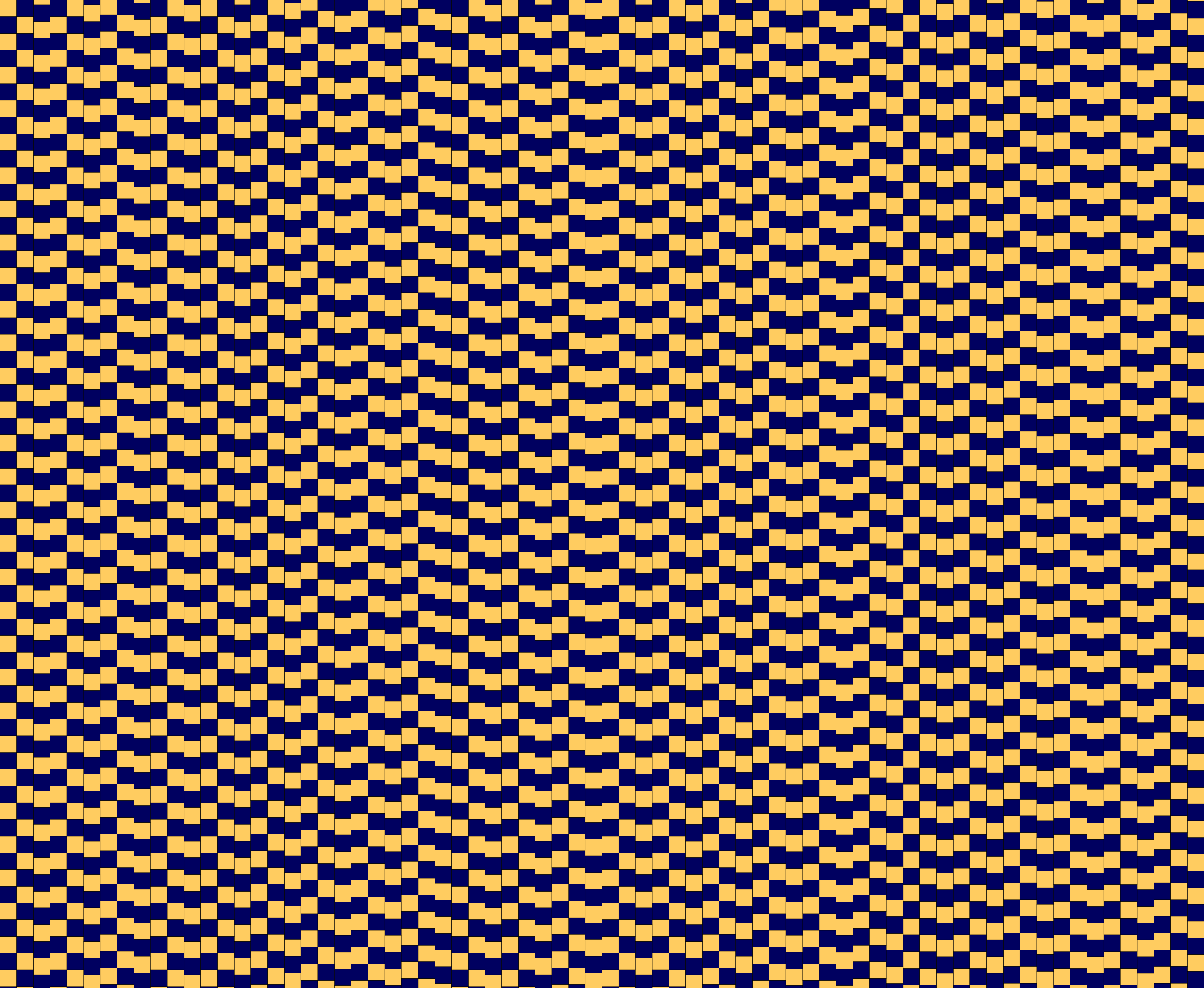download vectorformat Kenyon's non FLC