Kite Domino

• Finite Local Complexity
• MLD Class Pinwheel
• Polytopal Tiles
• Self-Similar Substitution
• With Decoration

Info

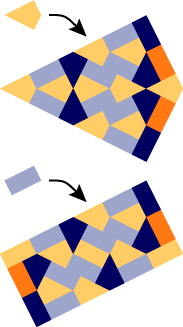
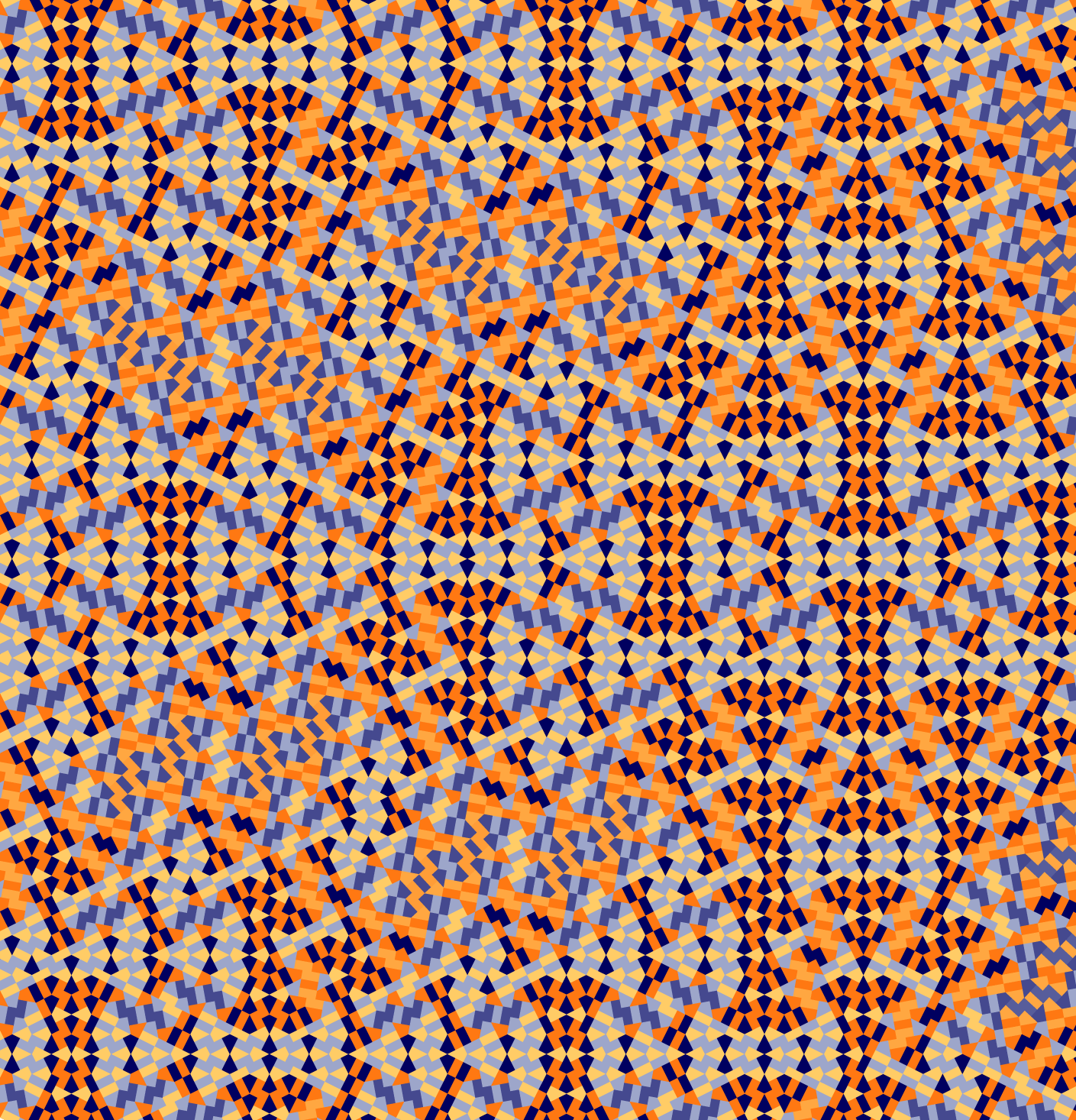
This is a variation of the pinwheel substitution. The kite-domino tilings are mld to the pinwheel tilings. The two prototiles are made of two pinwheel triangles, glued together at their long edge. There are two ways to do so, one gives a kite (a quadrilateral with edge lengths 1,1,2,2) and a domino (a rectangle with edge lengths 1,2,1,2). Then the substitution rule is obtained by considering two steps of the pinwheel substitution as one step. Thus, the inflation factor of the kite-domino tiling is 5. This version was introduced in [BFG07] . It is useful to determine the frequency of any patch in the original pinwheel tiling. In particular, it was used in [BFG07] to obtain the frequency module of all point pairs, which turned out to be ¹⁄₂₆₄ Z[¹⁄₅]. The matching rules for the pinwheel tiling, developed by C.Radin [Rad94] , can be used as matching rules for the kite domino tiling as well.

Substitution Rule

Patch

---

References

[BFG07]
Baake, M and Frettlöh, D and Grimm, U
A radial analogue of Poisson's summation formula with application to powder diffraction and pinwheel patterns
J. Geom. Phys 2007, 57, pp. 1331-1343,

[Rad94]
Radin, C
The pinwheel tilings of the plane
Ann. of Math. (2) 1994, 139, 3, pp. 661--702, 95d:52021

---