Peano Curve Substitution Tiling

• FASS-curve
• Finite Local Complexity
• Limitperiodic
• Polytopal Tiles
• Self-Similar Substitution
• With Decoration

Info

The Peano Curve is one the earliest known FASS-curves. The original algorithm in [pea1890] bases on one substitution rule and an additional rule which describes how the substitutes have to be connected. As briefly mentioned in [pau2021] it is also possible to create the Peano Curve by a substitution tiling with two substitution rules and appropriate decorations. The inflation factor $q$ is 3 and the lines are shifted slightly away from the center of the sides to illustrate the matching rules.

Substitution Rule

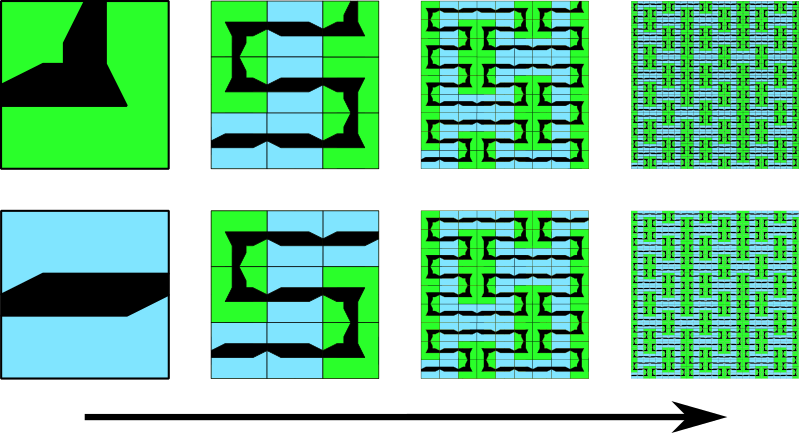
Patch

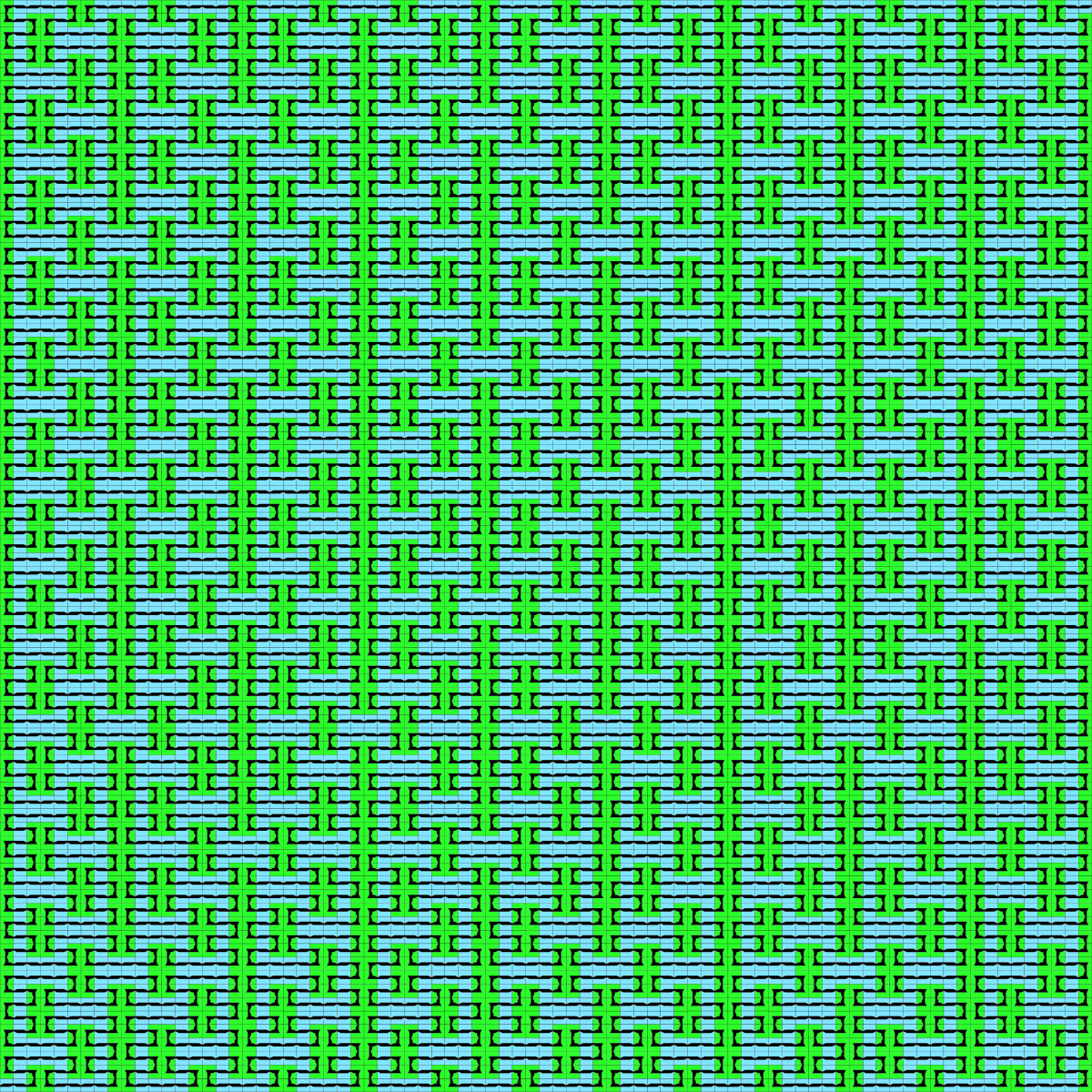download vectorformat Peano Curve Substitution Tiling

---

References

[Pau2021]
Pautze, S
Space-Filling, Self-Similar Curves of Regular Pentagons, Heptagons and Other n-Gons
Proceedings of Bridges 2021: Mathematics, Art, Music, Architecture, Culture 2021, 38, 157-164, bridges2021-157.pdf

[pea1890]
Peano, G
Sur une courbe, qui remplit toute une aire plane
Mathematische Annalen 1890, 36, 157-160, doi.org/10.1007/BF01199438

---