Chair

Info

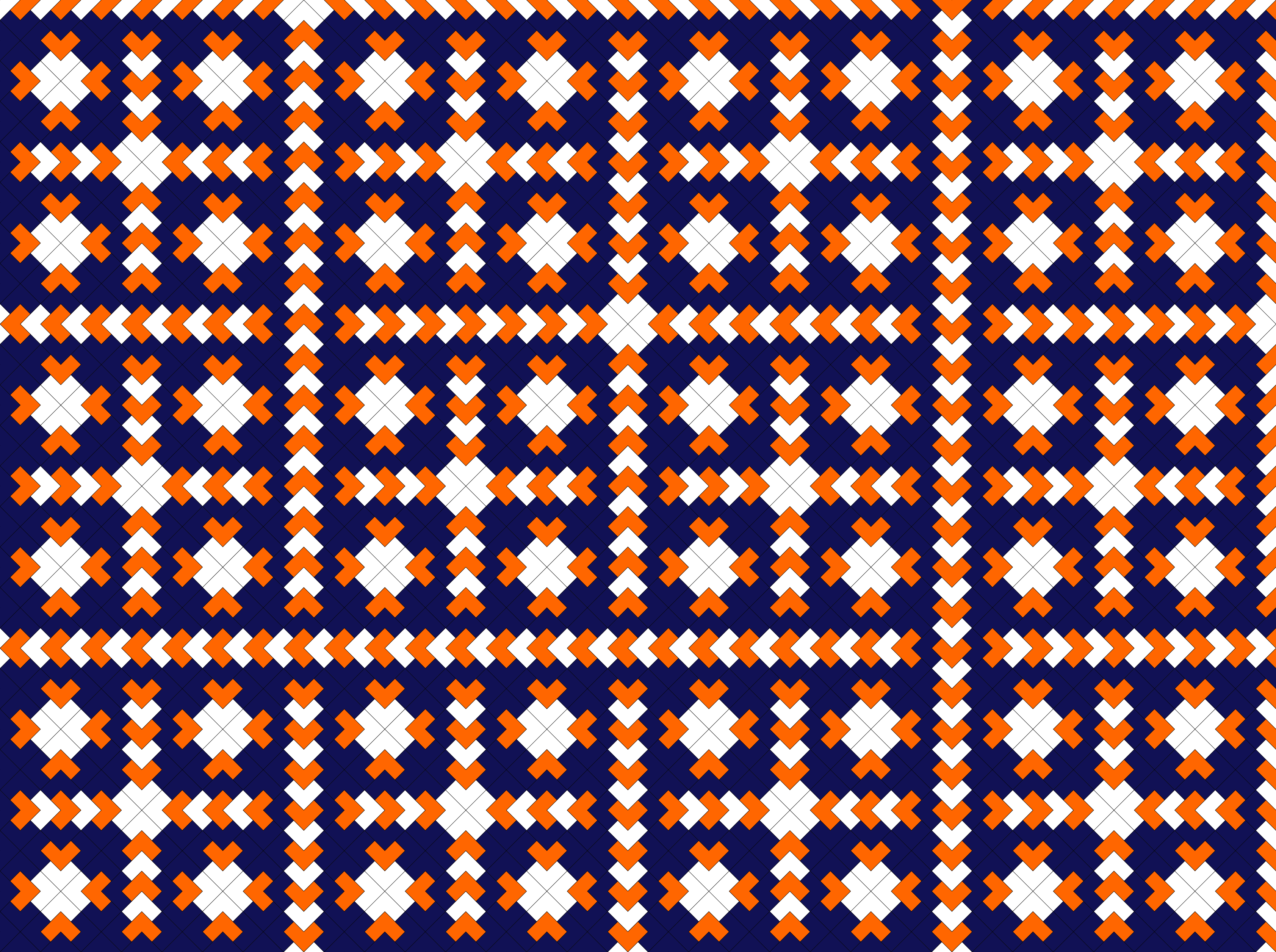
The chair tiling, as most tilings presented here, is nonperiodic. But there is a strong resemblance to periodic tiling. For instance, the set of vertex points in the tiling obviously spans a square lattice. Moreover, it is possible to detect large subsets in the tiling which are fully periodic. For instance, consider the pattern of white crosses (consisting of four tiles each) in the tiling. In fact, the chair tiling is the union of a countable set of fully periodic tile sets $L_{1}, L_{2}, L_{3}$…, where each $L_{i}$ possesses period vectors of length $2 \times 2^{i}$. This property is called limitperiodic, and it is the key to show how to obtain the chair tiling by a cut and project method with p-adic internal space, here: $\mathbb{Q}_2 \times \mathbb{Q}_2$. That was first carried out in [BaakeMS98] , see also [LMS03] . It is easy to see that there are no matching rules for the undecorated tiles. But [Goo99] found nice local matching rules with just two tiles, forcing tilings from which the chair tilings are locally derivable (see mld). Here, the tiles appear in three colours, depending on their relative position in the first-order super-tile. The colours do not mean that there are different substitution rules, all tiles are substituted in the same way.

Substitution Rule

Rule Chair

Patch

Patch Chair download vectorformat Chair

References

[BaakeMS98]
Baake, M and Moody, R V and Schlottmann, M
Limit-(quasi) periodic point sets as quasicrystals with p-adic internal spaces
Journal of Physics A: Mathematical and General 1998, 31(27), pp. 55-65, arxiv.9901008

[Goo99]
Goodman-Strauss, C
A small aperiodic set of planar tiles.
European J. Combin. 1999, 20, 5, pp. 375-384, MR1702375

[LMS03]
Lee, J E S and Moody, R V and Solomyak, B
Consequences of Pure Point Diffraction Spectra for Multiset Substitution Systems
Discrete and Computational Geometry 2003, 29, pp. 525-560, MR1702375